Lagrange’s Mean Value Theorem - 拉格朗日中值定理

Lagrange [lə'ɡrɑndʒ]：n. 拉格朗日 (法国著名数学家，力学家)
1

拉格朗日中值定理又称为拉氏定理，它反映了可导函数在闭区间上的整体的平均变化率与区间内某点的局部变化率的关系。

拉格朗日中值定理是罗尔中值定理的推广，同时也是柯西中值定理的特殊情形，是泰勒公式的弱形式 (一阶展开)。

1. Lagrange’s Mean Value Theorem - 拉格朗日中值定理

Lagrange’s mean value theorem (MVT) states that if a function $f\left(x\right)$ is continuous on a closed interval $\left[a,b\right]$ and differentiable on the open interval $\left(a,b\right)$, then there is at least one point $x=c$ on this interval, such that
拉格朗日中值定理 (MVT) 指出，如果函数 $f\left(x\right)$ 在闭合区间 $\left[a,b\right]$ 上是连续的，并且在开放区间 $\left(a,b\right)$ 上是可导的，那么在这个间隔上至少有一个点 $x=c$，这样

$$f\left(b\right)–f\left(a\right)=f^{\prime }\left(c\right)\left(b–a\right).$$

This theorem (First Mean Value Theorem) allows to express the increment of a function on an interval through the value of the derivative at an intermediate point of the segment.
该定理 (First Mean Value Theorem) 允许通过区间的中间点处的导数的值来表示函数在间隔上的增量。

如果函数 $f(x)$ 满足，
(1) 在闭区间 $[a,b]$ 上连续 ($f(x)$ is continuous in the closed interval $a\le x\le b$)
(2) 在开区间 $(a,b)$ 内可导 ($f(x)$ is differentiable in the open interval $a<x<b$)
那么在开区间 $(a,b)$ 内至少有一点 $c(a<c<b)$ 使等式 $f(b)-f(a)=f^{\prime }(c)(b-a)$ 成立。

Then according to Lagrange’s Theorem, there exists at least one point $c$ in the open interval $(a,b)$ such that:
然后根据拉格朗日定理，在开放区间 $(a,b)$ 中至少存在一个点 $c$，使得：
$$f^{\prime }(c)=\frac{f(b)-f(a)}{b-a}$$

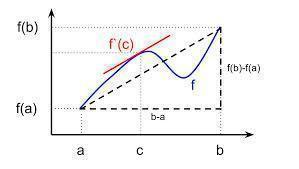
Lagrange’s theorem says that if there is a path between two points $A(a,f(a))$ and $B(b,f(a))$ in a 2-D plain then there will be at least one point $c$ on the path such that the slope of the tangent at point $c$, i.e., $(f^{\prime }(c))$ is equal to the average slope of the path, i.e., $f^{\prime }(c)=\frac{f(b)-f(a)}{b-a}$
拉格朗日定理表明如果在二维平面上两个点 $A(a,f(a))$ and $B(b,f(a))$ 之间存在一条路径，那么至少会有一个点 $c$ 在路径上，以使点 $c$ 处的切线斜率即 $(f^{\prime }(c))$ 等于路径的平均斜率，即 $f^{\prime }(c)=\frac{f(b)-f(a)}{b-a}$

Verify mean value theorm for $f(x)=x^2$ in interval $[2,4]$.
验证区间 $[2,4]$ 中 $f(x)=x^2$ 的均值定理。

First check if the function is continuous in the given closed interval, the answer is Yes. Then check for differentiability in the open interval $(2,4)$, Yes it is differentiable.
首先检查该函数在给定的闭区间内是否连续，答案为是。然后检查开区间 $(2,4)$ 中的可导性，是的，它是可导的。

$f^{\prime }(x)=2x$
$f(2)=4$ and $f(4)=16$

$\frac{f(b)-f(a)}{b-a}=\frac{16-4}{4-2}=6$

Mean value theorm states that there is a point $c\in (2,4)$ such that $f^{\prime }(c)=6$. But $f^{\prime }(x)=2x$ which implies $c=3$. Thus at $c=3\in (2,4)$, we have $f^{\prime }(c)=6$.
均值定理指出存在点 $c\in (2,4)$ 使得 $f^{\prime }(c)=6$，但是 $f^{\prime }(x)=2x$ 意味着 $c=3$。因此，在 $c=3\in (2,4)$，我们有 $f^{\prime }(c)=6$。